Relationship between Average Variable Cost (AVC) and Average Total Cost (ATC)
The Average Variable Cost (AVC) is a part of the Average Total Cost (ATC). So, it is given as;
ATC = AFC + AVC
Now, both AVC and ATC are “U” shaped curves, and the shape reflects the law of variable proportions. The minimum point of the ATC occurs to the right of the minimum point of the AVC. This is due to the fact that ATC includes AFC and AFC eventually falls continuously with increases in output.
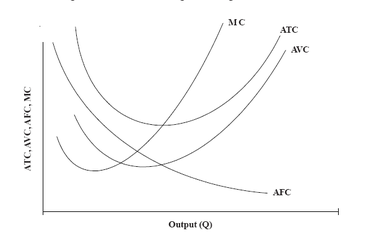
After the AVC has reached its lowest point and starts rising, its rise is over a certain range and is offset by a fall over that range despite the increase in AVC.
However, the rise in AVC eventually becomes greater than the fall in AFC so that the ATC starts increasing. The AVC approaches the ATC asymptotically as the output of X increases.
An asymptotic curve is that which is trying to touch or meet with the X-axis but it cannot meet.
In the diagram, the minimum AVC is reached at the point of intersection with AFC (say X1), while the ATC is at its minimum at the point of intersection with MC (say X2). So, between X1 and X2, the fall in AFC more than offsets the rise in AVC so that the ATC continues to fall.
Beyond X2, the increase in AVC is not offset or taken out of line by the fall in AFC, so that ATC rises.
Click here to save this Post as PDF
